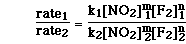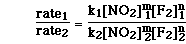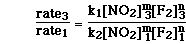Let's consider the following problem;
The following initial rate data were collected for
the reaction
2NO2(g) + F2(g) ---> 2NO2F(g)
at 100 degrees C.
|
Experiment #
|
[NO2]
|
[F2]
|
Initial Rate (M/min)
|
|
1
|
0.0482 M
|
0.0318 M
|
1.9 x 10-3
|
|
2
|
0.0120 M
|
0.0315 M
|
4.69 x 10-4
|
|
3
|
0.0480 M
|
0.127 M
|
7.57 x 10-3
|
i) Determine the reaction order for NO2(g)
and F2(g).
To determine the order with respect to NO2(g)
the ratio of experiments 1 and 2 must be used. Even though the concentration
of F2(g) is not exactly constant, it is to two significant figures.
The ratio of the two experiments can be written as;
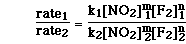
Since the rate constant is constant and the concentration
of F2(g) is constant we can cancel both of those terms and substitute
for the initial rate and concentration of NO2(g). We then have;

Reducing the two ratio yields;
4.05 = (4.02)m
1 = m
So the order of the reaction with respect to NO2(g)
is 1.
To determine the order with respect to F2(g)
the ratio of experiments 1 and 3 can be used. Even though the concentration
of NO2(g) is not exactly constant, it is to two significant figures.
The ratio of the two experiments can be written as;
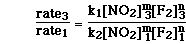
Since the rate constant is constant and the concentration
of NO2(g) is constant we can cancel both of those terms and substitute
for the initial rate and concentration of F2(g). We then have;

Reducing the two ratio yields;
3.98 = (3.99)n
1 = m
So the order of the reaction with respect to F2(g)
is 1.
ii) Determine the overall order of the reaction.
The overall order is just the sum of the order of the reaction with respect
NO2(g) and F2(g). So the overall order is equal to 2.