![]() Pre-lab Questions
Pre-lab Questions
![]() Experiment
Experiment
![]() Post-lab Questions
Post-lab Questions
EXPERIMENT 10: PROPERTIES OF WATER
The following preparatory questions should be answered before coming to class. They are intended to introduce you to several ideas important to aspects of the experiment. You must turn-in your work to your instructor before you will be allowed to begin the experiment. Be sure to bring a calculator and paper to laboratory.
![]()
1. Calculate the molality of glucose in a solution prepared by mixing 1.258 g C6H12O6 in
14.0 mL of water.
![]()
2. Briefly discuss the term freezing point depression.
![]()
3. (a) Complete the table.

3. (b) Why are molarity and molality more nearly equal for dilute solutions than for concentrated solutions?
![]()
![]()
3. (c) Under what conditions will molarity and molality of a solution be equal? Explain.
![]()
4. Why is it desirable to express concentration as molality rather than molarity when performing
experiments in which the temperature changes?
![]()
![]()
EXPERIMENT 10: PROPERTIES OF WATER
![]() Top
Top
EQUIPMENT:
| laboratory balance ...................... | 1 | 1 | |
| beaker, 100 mL ........................ | 1 | 1 | |
| beaker,600 mL ......................... | 1 | 1 | |
| glass rod................................. | 1 | 2 | |
| graduated cylinder, 25 or 50 mL ..... | 1 | 1 | |
| paper towels......................... | several | 1 |
It is suggested that students work in groups of two while collecting the experimental data.
The apparatus to be used in the following experiments is illustrated in Figure I (next page). The apparatus consists of an 18 x 150 mm test tube which will hold the solution whose freezing point is to be determined. The test tube is fitted with the two-holed rubber stopper. A thermometer is carefully inserted into the split hole of the rubber stopper and the wire stirrer is inserted through the other hole. A ring clamp is used to hold the test tube assembly, which is immersed in a ice/water/salt bath in a 600 mL beaker. A glass stirring rod is used to stir the ice/water/salt solution, while the wire stirrer is used mix the solution in the test tube. If you have any questions about the apparatus consult with your instructor.
Part I: The Freezing Point of a Pure Liquid
Add approximately 15 mL of deionized water to the 18 x 150 mm (3/4-inch) test tube.
Assemble the apparatus, with the test tube containing the water immersed in the ice-rock salt
mixture. (For best results use mostly ice & salt with just a little water.) The test tube should be
inserted deeply enough that the entire water sample is below the level of the ice-rock salt level in
the beaker. Stir both the water and the ice-rock salt solution continuously. Watch the mercury
level in the thermometer drop. The lowest constant temperature observed is the freezing point of
the water. When the temperature reaches a constant value, read the thermometer to the nearest 0.5
ºC.
NOTE: The solution may cool below the true equilibrium freezing point because there are no nucleation sites for solidification to occur. This is called supercooling. Stir the solution rapidly and the temperature will increase to the equilibrium freezing point and remain constant. (See Figure II - next page).
Obs. #1 Freezing Point of Pure Water _________________
Remove the test tube from the beaker. Dispose of the water sample and dry the test tube for later use.
![]()
![]()

![]()
Part II: The Freezing Point of Sucrose Solutions
Weigh a clean, dry 18 x 150 mm test tube, supported in a 100 mL beaker, using the laboratory
balance and record the mass below. Add between 15 and 16 mL of deionized water to the test tube.
Record the mass of the water+test tube+beaker below (Note: This mass is entered twice for ease of
calculations). Carefully add about 2.2 g of sucrose (C12H22O11) to the test tube and record the mass of
sucrose+water+test tube+beaker. After all of the sucrose has dissolved, assemble the apparatus with the
test tube containing the solution immersed in the ice-rock salt mixture. The test tube should be inserted
deeply enough that the entire solution sample is below the level of the ice-rock salt level in the beaker.
Stir both the sucrose solution in the test tube and the ice-rock salt solution continuously. Measure the
freezing point of this solution and record the temperature. Watch for supercooling!
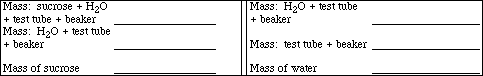
![]()
Calculate the molality of the sucrose solution.
Calc. #1
![]()
Obs. #2 Freezing Point of the Solution _______________
Obs. #3 Change in Freezing Point of Solution ___________ (Obs #1 - Obs #2)
Repeat the procedure above using about 4.4 g of sucrose. Record your data and observed freezing point below.
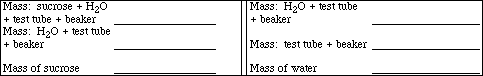
![]()
Calculate the molality of the sucrose solution.
Calc. #2
![]()
Obs. #4 Freezing Point of the Solution _______________
Obs. #5 Change in Freezing Point of the Solution _______ (Obs #1 - Obs #4)
![]()
![]()
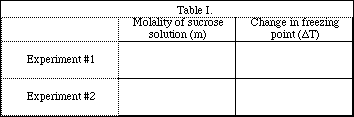
Complete the following table.
![]()
How did the freezing point change with change in the molality of the sucrose solution?
Expl. #1
![]()
Write a mathematical proportionality statement based on your data in Table I.
![]()
In order to make a proportionality into an equality, we need to incorporate a constant into
the equation. (For example, if a variable a is directly proportional to another variable b, we can
write a a b. To convert the proportionality statement to an equation we write a = kb, where k is a
proportionality constant.) We need an equation relating the molality of the solution to its change in
freezing point. We will call the required constant, Kf, the freezing point constant. Write the
equation obtained by including Kf in your proportionality statement. So that all answers in the
class will be consistent, be sure to place the constant on the "molality side" of the equation.
Calculate the magnitude of the freezing point depression constant based on your data. (Be sure to include the correct units for the constant.)
Calc #3
![]()
![]()
Part III: Freezing Point of Sodium Chloride Solutions
Weigh a clean, dry 18 x 150 mm test tube, supported in a 100 mL beaker, using the laboratory
balance and record the mass in the data sheet. Add between 15 and 16 mL of deionized water to the test
tube. Record the mass of the water+test tube+beaker on the data sheet. Carefully add about 0.5 g of
sodium chloride to the test tube and record the mass of sodium chloride+water+test tube+beaker. After
all of the sodium chloride has dissolved, assemble the apparatus with the test tube containing the solution
immersed in the ice-rock salt mixture. The test tube should be inserted deeply enough that the entire
solution sample is below the level of the ice-rock salt level in the beaker. Stir both the salt solution in the
test tube and the ice-rock salt solution continuously. Measure the freezing point of this solution and
record the temperature. Watch for supercooling!

Calculate the molality of the sodium chloride solution.
Calc. #4
![]()
Obs. #6 Freezing Point of the Solution _______________
Obs. #7 Change in Freezing Point of the Solution _______________
Repeat the procedure above using about 1.0 g of sodium chloride . Record your data and observed freezing point below.

Calculate the molality of the sodium chloride solution.
Calc. #5
![]()
Obs. #8 Freezing Point of the Solution _______________
![]()
Obs. #9 Change in Freezing Point of the Solution _______________
![]()
![]()
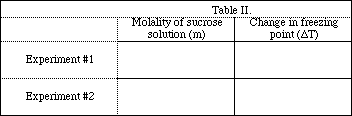
Complete the data Table.
![]()
How did the freezing point change with change in the molality of the sodium chloride solution?
Expl. #2
![]()
Write a mathematical proportionality statement based on your data in Table I.
![]()
In order to make a proportionality into an equality, we need to incorporate a constant into
the equation. (For example, if a variable a is directly proportional to another variable b, we can
write a a b. To convert the proportionality statement to an equation we write a = kb, where k is a
proportionality constant.) We need an equation relating the molality of the solution to its change in
freezing point. We will call the required constant, Kf, the freezing point constant. Write the
equation obtained by including Kf in your proportionality statement. So that all answers in the
class will be consistent, be sure to place the constant on the "molality side" of the equation.
Calculate the magnitude of the freezing point depression constant based on your data. (Be sure to include the correct units for the constant.)
Calc #6
![]()
![]()
Is there any difference between the constant obtained from Part II (sucrose solution) and the constant obtained in Part III (sodium chloride solution)? If so, how do they differ?
Expl. #3
![]()
What would you have to do to the constant from Part III to get close to the constant from Part II?
Expl. #4
![]()
Write the chemical equation for what occurs when sucrose is added to water.
Equ. #1
![]()
Write the chemical equation for what occurs when sodium chloride is added to water.
Equ. #2
![]()
For every mole of sucrose that dissolves, how many moles of 'particles' (sucrose molecules) are in
solution?
Expl. #5
![]()
For every mole of sodium chloride that dissolves, how many moles of 'particles' (ions) are in
solution?
Expl. #6
![]()
![]()
The equation
"DT = Kfm"
describes the freezing point depression of a solvent due to the addition of a nonvolatile solute. The freezing point constant, Kf, for a given solvent is constant and independent of the identity of the nonvolatile solute particles. In other words, the freezing point constant has to be the same in Part II and Part III of the experiment because water is the solvent in both parts. Explain the reason for the difference in the values obtained in Calc. #3 and Calc. #6.
Expl. #7
![]()
If the freezing point constant, Kf, is constant, on what does the freezing point of an aqueous
solution of a nonvolatile solute depend?
![]()
Expl. #8
![]()
![]()
These problems are to be turned in with the laboratory write-up. SHOW ALL CALCULATIONS.
1. Write a chemical equation which describes what happens when the following substances are added to water.
![]()
(a) C2H4(OH)2(l)
![]()
(b) Ca(NO3)2(s)
![]()
2. Complete the following table.
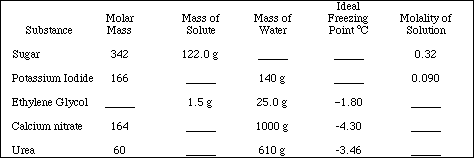
![]()
3. Calculate the boiling point of each of the solutions in Problem #2.
![]()
![]()
4. Freezing point depression is often used to experimentally determine the molecular mass of a solute, but boiling point elevation is rarely used. Considering the calculations you have just performed in questions #2 and #3, explain why this is so.
![]()
5. A solution of 0.684 g of chlorous acid, HClO2, in 100 g of water freezes at -0.24 ºC. Would
you classify HClO2 as a nonelectrolyte, a weak electrolyte, or a strong electrolyte?
![]()
Contents
![]() Return to
Return to![]() Index of Experiments
Index of Experiments